The 3D printing feature for creating free-form surface styling products opens up a magical magic box for us. Let the products of this world become more fun and brainstorming. Researchers at Carnegie Mellon have been working to improve new methods of building telescopic structures, especially along the direction of the curve. Recently, they created a new model based on mathematical algorithms. This modeling work that changes freely along the curve is presented in the form of a telescope.
Christopher Yu, Keenan Crane and Stelian Coros detailed their findings in their recently published "Structural Design for Telescopic Structures".
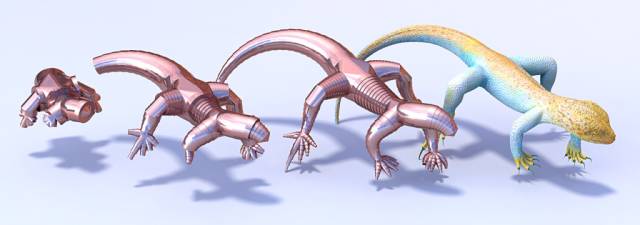
The telescoping structure is suitable for deploying applications that must be compact and changeable. However, to date, there has been no systematic study of the types of shapes that can be modeled by telescopic structures, nor the utilities for telescoping designs.
Carnegie Mellon's focus is on segmented spiral space curves and torsional pulses. On this basis, the team continues to develop scalable architectures for users. The user's sketches and grids are used to create curve skeletons, and the research team has expanded its use in animation and robotics.
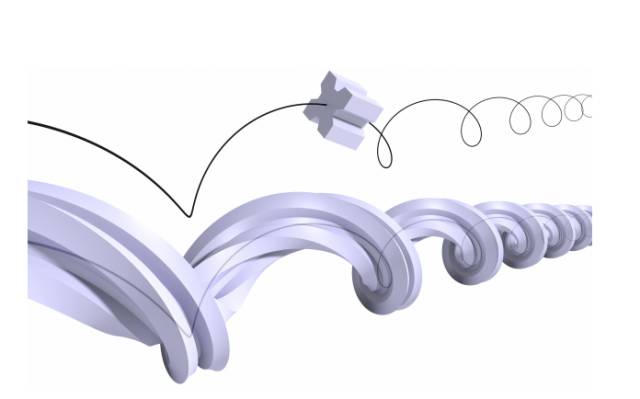
The basic shape of the telescopic shell is a spiral path created by the inner curve, sweeping the resulting solid. Any such shape (bottom) can achieve its own sliding through a continuous spiral motion.
Researchers point out that although there are not many studies on this topic, researchers in the aerospace industry have had the opportunity to use such modeling algorithms to develop applications such as robots and bridges. Previous work has focused on:
- deployable structure
- Compact storage
- Calculate the fold
- the geometry of the space curve
- Grid skeletonization
When using a segmented spiral curve, the team's goals are as follows:
- given as a densely sampled polyline
- Smoothing the curve by heat flow curvature
- Divide the curve into segments and determine the best approximation of the torsion by calculation
- Convert each segment into a telescopic shell
The team found that they were able to optimize by building curvature and twisting. You can avoid drift in the end of the curve (which may need to be connected to other curves) by finding the rotation and uniform scaling that aligns the endpoint of the spiral approximation with the endpoint of the given curve.
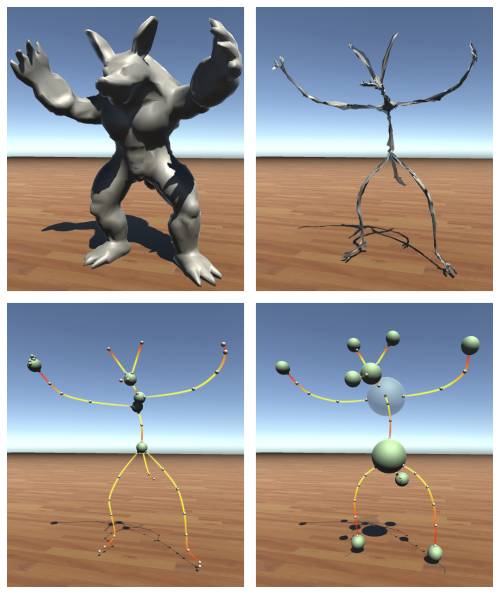
The team must also work hard to adjust the 3D printing method to get the desired result. They must create a linear tape with a shell radius to keep the shells connected and make each shell longer.

Next, the research team will continue their work on the basis of the current model, with improved work objectives including improved geometric approximation algorithms and the use of telescopic separators to replace some of the current joints.
The value of this type of modeling is that the mechanical actuation of the extension and torsion pulses provides automatic deployability and control for the mechanical field, making it suitable for applications in engineering and robotics.
(Editor)
Silicone Hookah,Silicone Hookah Smoking,Silicone Weed Pipe,Hookah Smoking Pipes Weed
Wenzhou Xinyu Imp & Exp Co.,Ltd , https://www.somgar.com